The number of atoms or molecules (n) in a mass (m) of a pure material having atomic or molecular weight (M) is easily computed from the following equation using Avogadro's number (NA = 6.022×10 23 atoms or molecules per gram-mole): M mN n A (1) In some situations, the atomic number density (N), which is the concentration of atoms or molecules per. ATOMIC MASS.TO CALCULATE THE ATOMIC MASS OF AN ELEMENT, MULTIPLY THE MASS OF EACH ISOTOPE BY ITS NATURAL ABUNDANCE, EXPRESSED AS A DECIMAL, AND THEN ADD THE PRODUCTS. Rekordbox 30 day trial. SAMPLE PROBLEM 1.RUBIDIUM HAS TWO COMMON ISOTOPES, 85-RB AND 87-RB.
Imagine that you have a pile of rocks to move, and need to decide what equipment to rent so that you can move them. If the rocks are fairly small, you can get a shovel to pick them up. Larger rocks could be moved by hand, but big boulders will need some sort of mechanical scoop. The amount of each kind of rock will also determine how much time you will need to get the job done. Knowing the relative amounts of large, medium, and small rocks can be very useful in deciding how to approach the job.
- Average atomic masses listed by IUPAC are based on a study of experimental results. Bromine has two isotopes, 79 Br and 81 Br, whose masses (78.9183 and 80.9163 amu) and abundances (50.69% and 49.31%) were determined in earlier experiments. Calculate the average atomic mass of Br based on these experiments.
- Atomic mass can be defined as the total mass of one atom of any given element. The unit of atomic mass is called the unified atomic mass unit (denoted by ‘u’). Most of the atomic mass of a substance is made up of protons and neutrons. Therefore, it is almost equal to its mass number.
- The standard for one atomic mass unit is equal to 1/12th of the mass of a standard carbon-12 isotope. Atomic mass units tell the mass of one mole of a given element or molecule in grams.
Percent Natural Abundance
Most elements occur naturally as a mixture of two or more isotopes. The table below shows the naturally occuring isotopes of several elements, along with the percent natural abundance of each.
| Element | Isotope (Symbol) | Percent Natural Abundance | Atomic Mass (left( text{amu} right)) | Average Atomic Mass (left( text{amu} right)) |
|---|---|---|---|---|
| Hydrogen | (ce{_1^1H}) | 99.985 | 1.0078 | 1.0079 |
| (ce{_1^2H}) | 0.015 | 2.0141 | ||
| (ce{_1^3H}) | negligible | 3.0160 | ||
| Carbon | (ce{_6^{12}C}) | 98.89 | 12.000 | 12.011 |
| (ce{_6^{13}C}) | 1.11 | 13.003 | ||
| (ce{_6^{14}C}) | trace | 14.003 | ||
| Oxygen | (ce{_8^{16}O}) | 99.759 | 15.995 | 15.999 |
| (ce{_8^{17}O}) | 0.037 | 16.995 | ||
| (ce{_8^{18}O}) | 0.204 | 17.999 | ||
| Chlorine | (ce{_{17}^{35}Cl}) | 75.77 | 34.969 | 35.453 |
| (ce{_{17}^{37}Cl}) | 24.23 | 36.966 | ||
| Copper | (ce{_{29}^{63}Cu}) | 69.17 | 62.930 | 63.546 |
| (ce{_{29}^{65}Cu}) | 30.83 | 64.928 |
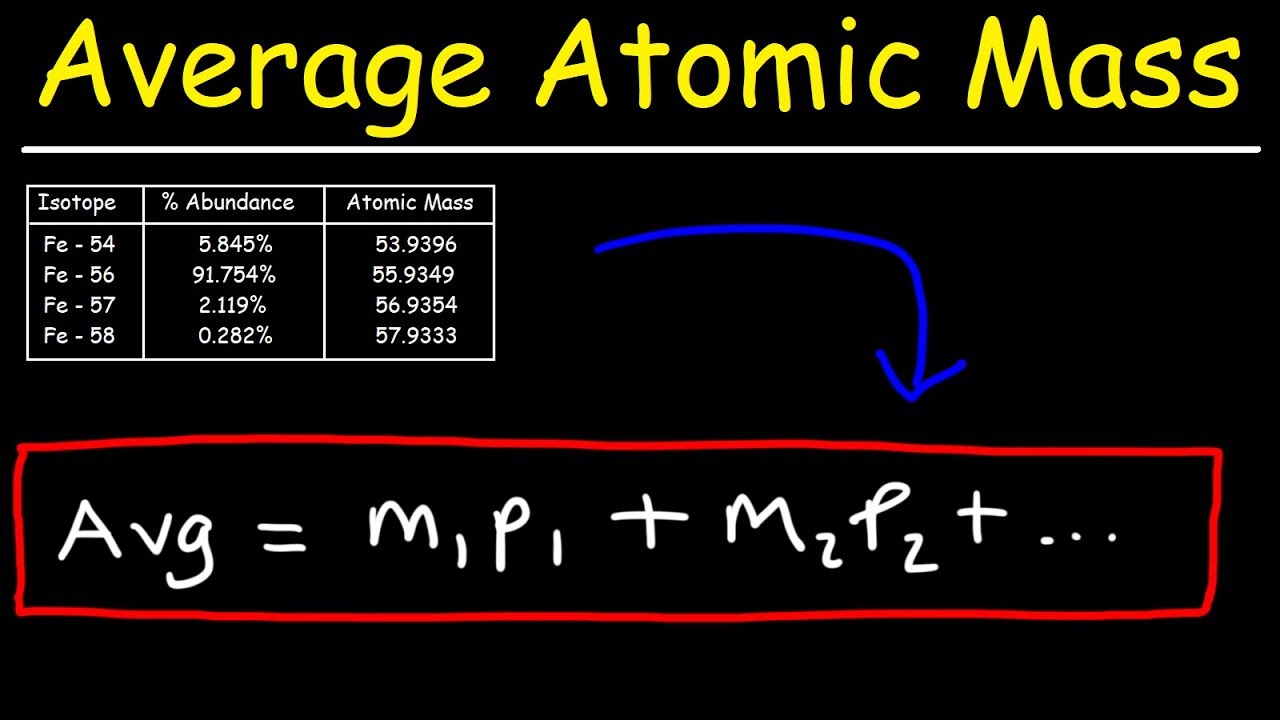
For some elements, one particular isotope predominates greatly over the other isotopes. Naturally occurring hydrogen is nearly all hydrogen-1 and naturally occurring oxygen is nearly all oxygen-16. For many other elements, however, more than one isotope may exist in more substantial quantities. Chlorine (atomic number 17) is a yellowish-green toxic gas. About three quarters of all chlorine atoms have 18 neutrons, giving those atoms a mass number of 35. About one quarter of all chlorine atoms have 20 neutrons, giving those atoms a mass number of 37. Were you to simply calculate the arithmetic average of the precise atomic masses, you would get 36.
[frac{left( 34.969 + 36.966 right)}{2} = 35.968 : text{amu}]
Clearly the actual average atomic mass from the last column of the table is significantly lower. Why? We need to take into account the percent natural abundance of each isotope in order to calculate what is called the weighted average. The atomic mass of an element is the weighted average of the atomic masses of the naturally occurring isotopes of that element. The sample problem below demonstrates how to calculate the atomic mass of chlorine.
Example (PageIndex{1})
Use the atomic masses of each of the two isotopes of chlorine along with the respective percent natural abundance to calculate the average atomic mass of chlorine.
Solution
Step 1: List the known and unknown quantities and plan the problem.
Known

- Chlorine-35: atomic mass (= 34.969 : text{amu}) and percent abundance (= 75.77%)
- Chlorine-37: atomic mass (= 36.966 : text{amu}) and percent abundance (= 24.23%)
Unknown
- Average atomic mass of chlorine
Change each percent abundance into decimal form by dividing by 100. Multiply this value by the atomic mass of that isotope. Add together for each isotope to get the average atomic mass.
Step 2: Calculate.
[begin{array}{ll} text{chlorine-35} & 0.7577 times 34.969 = 26.50 : text{amu} text{chlorine-37} & 0.2423 times 36.966 = 8.957 : text{amu} text{average atomic mass} & 26.50 + 8.957 = 35.45 : text{amu} end{array}] Addictive drums 2.
Note: Applying significant figure rules results in the (35.45 : text{amu}) result without excessive rounding error. In one step:
[left( 0.7577 times 34.969 right) + left(0.2423 times 36.966 right) = 35.45 : text{amu}]
Step 3: Think about your result.
The calculated average atomic mass is closer to 35 than to 37 because a greater percentage of naturally occurring chlorine atoms have the mass number of 35. It agrees with the value from the table above.
Summary
- The atomic mass of an element is the weighted average of the atomic masses of the naturally occurring isotopes of that element.
- Calculations of atomic mass use the percent abundance of each isotope.
Contributors and Attributions
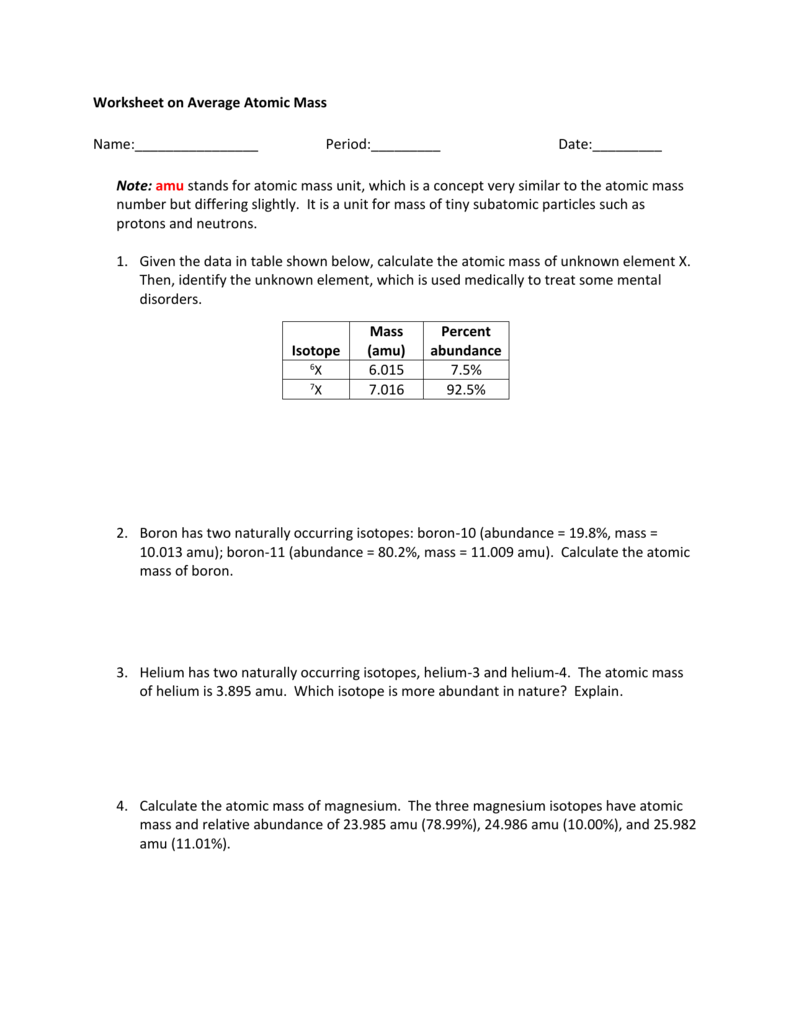
Calculating Atomic Mass Worksheet Answers
CK-12 Foundation by Sharon Bewick, Richard Parsons, Therese Forsythe, Shonna Robinson, and Jean Dupon.
